To determine the charge on an electron, Millikan sprayed tiny oil droplets into the top compartment of a drum. Here is an illustration of the drum and its compartments:

As you can see, the drum consists of a top and middle compartment. These compartments are separated by two plates. The top plate is positively charged and has a hole drilled in its center, while the bottom plate is negatively charged without a hole drilled in its center. As Millikan sprayed tiny oil droplets into the top compartment, some were attracted and fell through the hole into the middle compartment. As they fell through, Millikan fired x-rays into the middle compartment. These x-rays knocked out electrons from surrounding air molecules in the compartment. These electrons were then attracted to and settled on the oil droplets, turning them into charged oil droplets. Millikan then adjusted the electric field between the top and middle plate until such a point that the electrostatic pull on the droplet was equal to the gravitational pull. At this point, the droplet appeared suspended in the compartment. Millikan then applied the equations describing these forces to calculate the charge on the droplets. From the calculations, he found that different droplets had different charges. However, these charges were always a multiple of the smallest charge. This smallest charge had a value of 1.60 x 10-19C, where C stands for Coulombs, the unit for electric charge. From this, Millikan assumed that the 1.60 x 10-19C was the charge on an electron.
Can we use the charge of an electron to calculate its mass?
Yes, we can. If you recall, Thomson used his cathode ray tube experiment to determine the mass-to-charge ratio of an electron. Using this ratio and the charge on an electron, we can calculate its mass. Here is how:
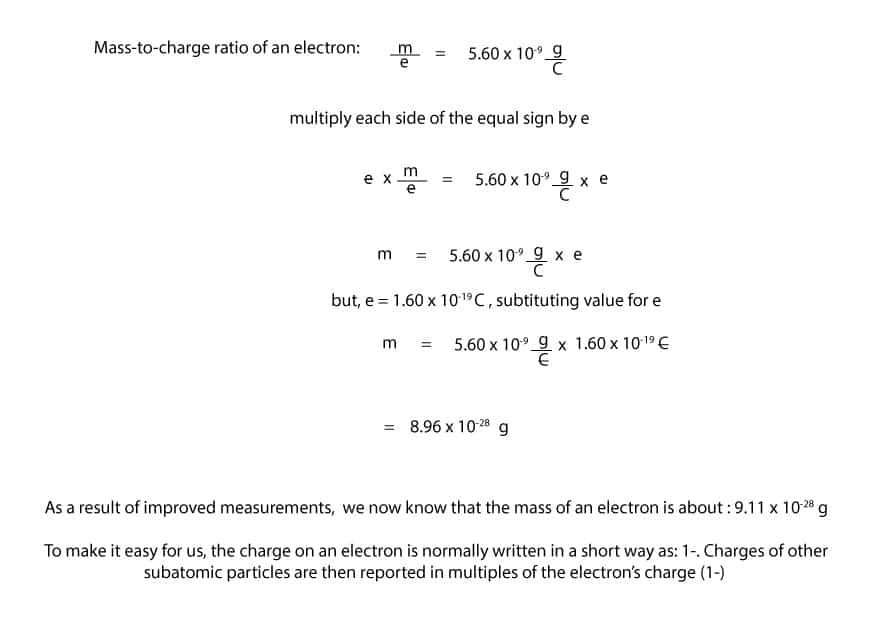
As you can see from above, to calculate the mass of an electron, you must multiply the mass-to-charge ratio by the charge of an electron.
To learn about the properties of subatomic particles, click here