Stoichiometry is a measure that uses the coefficients in a balanced chemical equation and the mole concept to relate quantities of reactants and products to each other.
Stoichiometry is based on the law of conservation of mass. The law of conservation of mass says that the mass of reactants before reaction is always equal to the mass of the products after the reaction. Reactants refer to the starting chemicals in the reaction, while products refer to the chemicals formed from atoms of the reactants when these atoms regroup in new ways. Because the mass of these atoms remains unchanged before and after the reaction, mass is always said to be conserved. In contrast, in nuclear reactions some matter is converted to energy, as a result mass is not conserved.
The concepts involved in stoichiometry are widely used in chemistry. For instance, let’s say at your chemical company you want to combine X and Y amounts of reactants to make product Z. To figure out how much product Z you can theoretically produce, you will apply stoichiometry. That is first, you will determine the limiting reactant by converting the mass or volume of your reactants to moles. Once the limiting reactant is determined, you will use the mole-ratio between limiting reactant and product in the balanced chemical equation to convert moles of the limiting reactant to moles of the product. Once you get moles of the product, you will multiply moles of product by the product molar mass to determine the theoretical yield of product Z.
Stoichiometry calculations appear in different forms. They can be
- Mass-to-mass stoichiometry problems
- Mass-to-volume stoichiometry problems
- Volume-to-volume stoichiometry problems
- Volume-to-mass stoichiometry problems.
Here is a concept map showing the relationships between the different forms of stoichiometry problems.
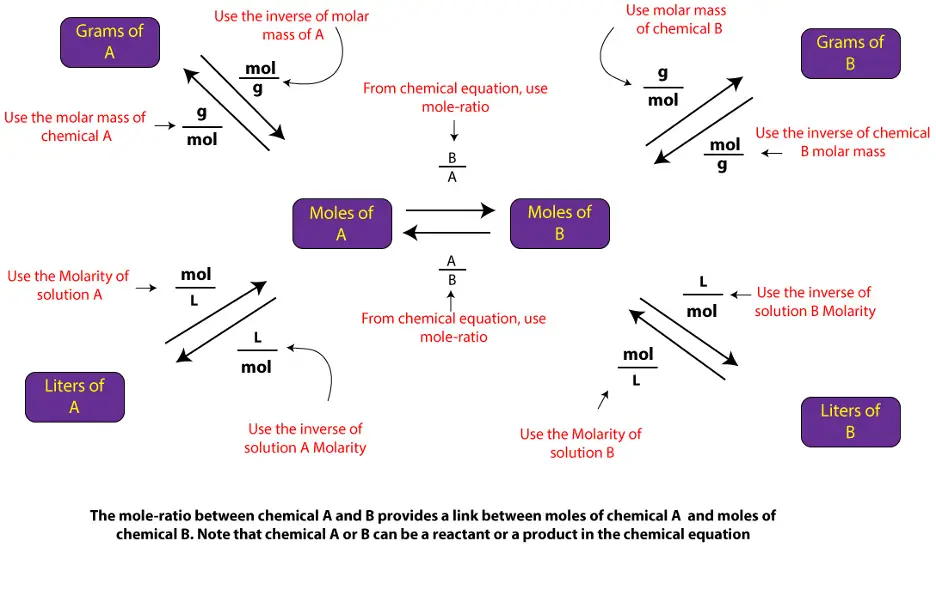
As you can see in the map, all the different forms of stoichiometry problems, go through moles. So, understanding the mole concept and how to apply it to write mole-mole ratio from a balanced chemical equation is critical to solving stoichiometry problems.
From the map, you can see that to go from mass of chemical A to mass of chemical B, you must first use molar mass of compound A to convert mass of chemical A to moles of chemical A, and then use the mole-ratio from balanced chemical equation to convert moles of chemical A to moles of chemical B. Once you get moles of chemical B, you can proceed in two ways. First, you can multiply moles of chemical B by its molar mass to get mass in grams of chemical B. Or you can multiple moles of chemical B by the inverse of Molarity to convert moles of chemical B to volume of chemical B
Similarly, to go from volume in liters to mass in grams, first, you will use the concentration in Molarity to convert volume in liters of chemical A to moles of chemical A, and then use the mole-ratio from balanced chemical equation to convert moles of chemical A to moles of chemical B. Once you get moles of chemical B, you can proceed in two ways. First, you can multiply moles of chemical B by its molar mass to get mass in grams of chemical B. Or you can multiple moles of chemical B by the inverse of Molarity to convert moles of chemical B to volume of chemical B.
Similarly, the other paths on the map can be described as such.
Here is another concept map showing relationships in gas stoichiometry
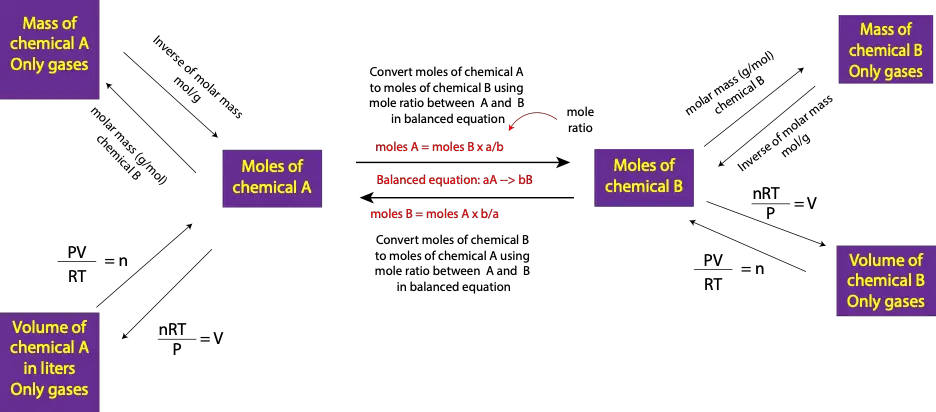
As you can see, it’s like the previous map, however in gas stoichiometry you rely on gas laws or the ideal gas equation to convert volume of gas A in liters to moles of gas A. To practice, use both concept maps as blueprint to solve stoichiometry problems.
